Discussion (with examples) on integration techniques is complete! (As usual, I welcome comments and info about typos, dead links, etc.)
Equations and inequalities- Curves and transformations
- Functions
- Arithmetic and geometric progressions
- Series and the sigma notation
- Differentiation
- Maclaurin series and binomial expansion
Integration techniques- Definite integrals
- Differential equations
- Vectors
- Complex numbers
Next up: randomly generated question on integration to test your concepts. Then vectors, which will likely take a whole chunk of time and effort. Let’s work hard together!
Picture credit: Pixabay and geralt .
Some further discussion for those interested:
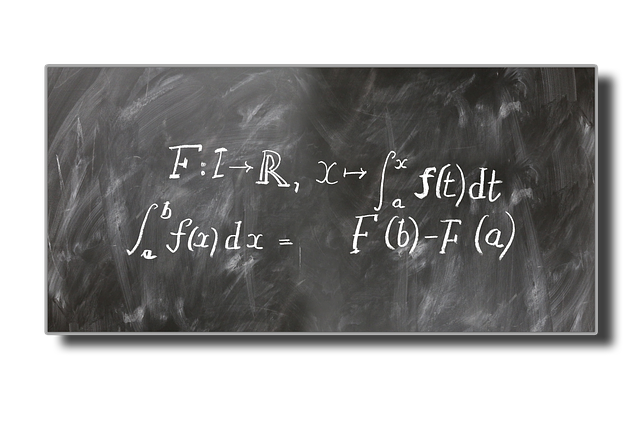
When we write $\int f(x) \; \mathrm{d}x$, we call the result the (indefinite) integral of $f(x)$. At higher levels, we typically denote our result by the expression “$F(x)$” and call it the “anti-derivative” of $f(x)$. Integration, on the other hand, refers to a process of taking the limits of a special type of sum (at least in the context of Riemann integrals). We will see a glimpse of this in chapter 9. A beautiful result, called the “fundamental theorem of calculus”, says that the processes of differentiation and integration are opposites (with a few technical qualifiers). In other words, integration is no different from anti-differentiation: the naming convention we adopt is still correct (albeit with quite a bit swept under the carpet).